In mathematics, an embedding (or imbedding) is one instance of some mathematical object contained within another instance, such as a group that is a subgroup.

Contents

1 Topology/Geometry 1.1 General topology 1.2 Differential geometry 1.3 Riemannian geometry 2 Algebra 2.1 Field Theory 3 Domain theory 4 See also

Topology/Geometry

General topology

In general topology, an embedding is a homeomorphism onto its image. More explicitly, a map f : X → Y between topological spaces X and Y is an embedding if f yields a homeomorphism between X and f(X) (where f(X) carries the subspace topology inherited from Y). Intuitively then, the embedding f : X → Y lets us treat X as a subspace of Y. Every embedding is injective and continuous. Every map that is injective, continuous and either open or closed is an embedding; however there are also embeddings which are neither open nor closed. The latter happens if the image f(X) is neither an open set nor a closed set in Y.

Differential geometry

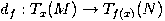In differential geometry: Let M and N be smooth manifolds and be a smooth map, it is called an immersion if for any point the differential is injective (here T_x(M) denotes tangent space of M at x). Then embedding, or smooth embedding is defined to be an immersion which is an embedding in the above sense (i.e. homeomorphism onto its image). When the manifold is compact, the notion of smooth embedding, is equivalent to that of injective immersion.

In other words, embedding is diffeomorphism to its image, in particular the image of embedding must be a submanifold. Immersion is a local embedding (i.e. for any point there is a neighborhood such that is an embedding.)

An important case is N=Rⁿ. The interest here is in how large n must be, in terms of the dimension m of M. The Whitney embedding theorem states that n = 2m is enough. For example the real projective plane of dimension 2 requires n = 4 for an embedding. The less restrictive condition of immersion applies to the Boy's surface—which has self-intersections.

Riemannian geometry

In Riemannian geometry: Let (M,g) and (N,h) be Riemannian manifolds. An isometric embedding is a smooth embedding f : M → N which preserves the metric in the sense that g is equal to the pullback of h by f, i.e. g = f*h. Explicitly, for any two tangent vectors

we have

g(v,w) = h(df(v),df(w)).

Analogously, isometric immersion is an immersion between Riemannian manifolds which preserves the Riemannian metrics.

Equivalently, an isometric embedding (immersion) is a smooth embedding (immersion) which preserves length of curves (cf. Nash embedding theorem).

Algebra

Field Theory

In field theory, an embedding of a field E in a field F is a ring homomorphism σ : E → F.

The kernel of σ is an ideal of E which cannot be the whole field E, because of the condition σ(1)=1. Therefore the kernel is 0 and thus any embedding of fields is a monomorphism. Moreover, E is isomorphic to the subfield σ(E) of F. This justifies the name embedding for an arbitrary homomorphism of fields.

Domain theory

In domain theory, an embedding of partial orders is F in the function space [X → Y] such that

1. For all x₁, x₂ in X, x₁ ≤ x₂ if and only if F (x₁) ≤ F(x₂) and
2. For all y in Y, {x : F (x) ≤ y } is directed.

Based on an article from FOLDOC, used by permission.

See also

• Inclusion (mathematics)