Electron configuration is the arrangement of electrons in an atom, molecule or other body. Specifically, it is the placement of electrons into atomic, molecular, or other forms of electron orbitals.
Electrons are fermions and are thus subject to the Pauli exclusion principle, which states that no two fermions can occupy the same quantum state at once. This is the fundamental basis of the configuration of electrons in an atom: once a state is occupied by an electron, the next electron must occupy a different quantum mechanical state.
The spherical part of the decomposition of the Schrödinger equation for an atom bears remarkable similarity to the equations of motion of a particle in a circular orbit about an attracting potential (in this case, the nucleus). Thus it should be no surprise that the solutions can be categorized in terms of their angular momentum. The solutions to the spherical compnent of the Schroedinger equation are given by the spherical harmonics. These solutions are described by two quantum numbers, l and m. Here, l is an integer, greater than or equal to zero, and m, also an integer, is bounded:  ; thus m can take on 2l+1 different values. The total angular momentum (the absolute value of the angular momentum) is given by
; thus m can take on 2l+1 different values. The total angular momentum (the absolute value of the angular momentum) is given by  , where
, where 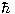 is Planck's constant. Thus one says that l corresponds to the angular momentum of the state. The various states relating to different values of l are sometimes called sub-shells, and (mainly for historical reasons) are referred to by letter, as follows:
is Planck's constant. Thus one says that l corresponds to the angular momentum of the state. The various states relating to different values of l are sometimes called sub-shells, and (mainly for historical reasons) are referred to by letter, as follows:
| l value |
Letter |
Maximum number of electrons in shell =2*(2*l+1) |
| 0 |
s |
2=2*(2*0+1) |
| 1 |
p |
6=2*(2*1+1) |
| 2 |
d |
10=2*(2*2+1) |
| 3 |
f |
14=2*(2*3+1) |
| 4 |
g |
18=2*(2*4+1) |
Historical note: The names 's', 'p', 'd', and 'f' originate from a now-disused system of categorizing spectral lines as "sharp", "principal", "diffuse", or "fundamental". When the first four types of orbitals were described, they were associated with these spectral line types, but there were no other names. The designations 'g' and 'h' were derived by following alphabetical order.
Each of the different angular momentum states can take 2(2l + 1) electrons. This is because the third quantum number ml (which can be thought of [somewhat inaccurately] as the [quantised] projection of the angular momentum vector on the z-axis) runs from −l to l in integer units, and so there are 2l + 1 possible states. Each distinct nlml state can be occupied by two electrons with opposing spins (given by the quantum number ms), giving 2(2l + 1) electrons overall. States with higher l than given in the table are perfectly permissible in theory, but these values cover all ground state atoms so far discovered.
For a given value of n the possible values of l range from 0 to n − 1; therefore, the n = 1 shell only possesses an s subshell and can only take 2 electrons, the n = 2 shell possesses an s and a p subshell and can take 8 electrons overall, the n = 3 shell possesses s, p and d subshells and has a maximum of 18 electrons, and so on (generally speaking, the maximum number of electrons in the nth energy level is 2n2). The following calculation yields exactly this result:
 .
.
In the ground state of an atom, the states are "filled" in order of increasing energy; i.e., the first electron goes into the lowest energy state, the second into the next lowest, and so on. The fact that the 3d state is higher in energy than the 4s state but lower than the 4p is the reason for the existence of the transition metals. The order in which the states are filled is as follows:
1s
2s 2p
3s 3p
4s 3d 4p
5s 4d 5p
6s 4f 5d 6p
7s 5f 6d 7p
8s 5g 6f 7d 8p
...
This leads directly to the structure of the periodic table. The chemical properties of an atom are largely determined by the arrangement of the electrons in its outermost ("valence") shell (although other factors, such as atomic radius, atomic mass, and increased accessibility of additional electronic states also contribute to the chemistry of the elements as atomic size increases).
Progressing through a group from lightest element to heaviest element, the outer-shell electrons (those most readily accessible for participation in chemical reactions) are all in the same type of orbital, with a similar shape, but with increasingly higher energy and average distance from the nucleus. For instance, the outer-shell (or "valence") electrons of the first group, headed by hydrogen all have one electron in an s orbital. In hydrogen, that s orbital is in the lowest possible energy state of any atom, the first-shell orbital (and represented by hydrogen's position in the first period of the table). In francium, the heaviest element of the group, the outer-shell electron is in the seventh-shell orbital, significantly further out on average from the nucleus than those electrons filling all the shells below it in energy. As another example, both carbon and lead have four electrons in their outer shell orbitals.
Because of the importance of the outermost shell, the different regions of the periodic table are sometimes referred to as periodic table blocks, named according to the sub-shell in which the "last" electron resides, e.g. the s-block, the p-block, the d-block, etc.
An example of the notation commonly used to give the electron configuration of an atom, in this case silicon (atomic number 14), is as follows: 1s2 2s2 2p6 3s2 3p2 The numbers are the shell number, n; the letters refer to the angular momentum state, as given above, and the superscripted numbers are the number of electrons in that state for the atom in question. An even simpler version is simply to quote the number of electrons in each shell, eg (again for Si): 2-8-4.
In molecules, the situation becomes much more complex: see molecular orbitals for details. Similar, but not identical, arguments can be applied to the protons and neutrons in the atomic nucleus: see the shell model of nuclear physics.
There are two major ways to write an electron configuration. One is writing out the whole configuration, as in the example 1s2 2s2 2p6 3s2 3p2. The other is a shorthand using noble gases. An example of this would be argon, which is [Ne]3s23p6.
See also